REVIEW: Dielectric and Photoelectric Properties of Photosynthetic Reaction Centers
C. S. Chamorovsky1, S. K. Chamorovsky2, and A. Yu. Semenov1*
1Belozersky Institute of Physico-Chemical Biology and 2Department of Biophysics, Faculty of Biology, Lomonosov Moscow State University, Moscow, Russia; fax: (7-095) 939-3181; E-mail: semenov@genebee.msu.su* To whom correspondence should be addressed.
Received July 27, 2004
A brief review of studies of dielectric and photoelectric properties of photosynthetic reaction centers of purple bacteria as well as photosystem I and photosystem II of cyanobacteria and higher plants is given. A simple kinetic model of the primary processes of electron transfer in photosynthesis is used to discuss possible mechanisms of correlation between rate constant of charge transfer reaction, free energy of electron transition, and effective dielectric constant in the locus of corresponding carriers.
KEY WORDS: photosynthetic reaction center complexes, photosynthetic bacteria, photosystems I and II, cyanobacteria, electron transfer, membrane potential, dielectric constant
The processes of charge separation in photosynthetic reaction centers (RC) and further transfer of electron and proton along the photosynthetic chain are accompanied by generation of electric potential (Deltapsi), which can be detected using instrumental methods. The direct electrometric method suggested in our laboratory at the Belozersky Institute of Physico-Chemical Biology provided the opportunity to detect the rise-time of Deltapsi within the range from 200 nsec to 100 msec [1]. This method was used to study bacteriorhodopsin [2], bacterial photosynthetic reaction centers (BRC) [3], as well as photosystem (PS) I [4, 5] and PS II [6] of cyanobacteria.
Because the photoelectric signal amplitude measured by this method is proportional to dielectrically weighted distances, comparison of projections of the distance vectors between redox cofactors onto the membrane normal with the relative photovoltage amplitudes provides an opportunity to estimate the dielectric constant value (epsilon) at the corresponding chain segment.
Strictly speaking, dielectric constant is a macroscopic characteristic determined by the atomic state of the surrounding medium. This value can be calculated from the classical equation of electrostatics:
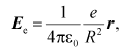 (1)
(1)where Ee is the strength of the electric field generated by an electron at the locus of a given atom in vacuum; epsilon0 is the dielectric permittivity of vacuum; e is electron charge; r is unity radius-vector connecting atom and electron; R is the distance between the atom and the electron.
Local (i.e., determined on the time scale of corresponding reaction) value of dielectric constant (epsiloneff) can be calculated from Eq. (2) derived in [7, 8]:
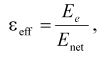 (2)
(2)where Enet is the net electric field strength at the chromophore locus with regard to the effect of charge screening.
It was demonstrated in various model systems, including genetically modified proteins (for example, barnase) that the macroscopic estimates of dielectric parameters did not change drastically on the angstrom scale [9, 10].
Photoelectric and dielectric properties of PS I were considered in our earlier reviews [11, 12]. The goal of this work was to review photoelectric and dielectric properties of BRC and PS II and to discuss possible mechanisms of correlation between values of charge transfer reaction rate constant and dielectric permittivity of photosynthetic pigment-protein complexes noted in the preceding works [11, 12]. The results of X-ray diffraction analysis of crystals of the protein complex of photosynthetic RC were from the Brookhaven Protein Databank (http://www.rcsb.org/pdb). Distances between centers of molecules were measured using the HyperChem 7 Professional software. The aggregate of the resulting estimates is the profile of distribution of effective dielectric constant along the electron transfer chain in the pigment-protein complex.
BACTERIAL REACTION CENTERS AND PHOTOSYSTEM I
Although the PS I complex differs significantly from the RC of purple photosynthetic bacteria, there are features of remarkable similarity between PS I and BRC, especially in the RC core and the donor sites. The estimates of the effective dielectric constant values for the BRC of Blastochloris (formerly Rhodopseudomonas) viridis are given in Table 1. It was shown in our earlier studies that electrogenic reduction of the photooxidized bacteriochlorophyll dimer P960 by the immediate electron donor, high-potential cyt c559, and further electron transfer from the second high-potential cyt c556 to oxidized cyt c559 account for 15 and 5% of the overall photoresponse amplitude Deltapsi, respectively [13]. The same relative photovoltages and effective dielectric constant values were obtained for the donor sites of the Rhodospirillum rubrum and Rhodobacter sphaeroides BRC [14].
Table 1. Calculation of effective dielectric
constant values in the protein domains between redox cofactors in
bacterial photosynthetic reaction centers of Blastochloris
viridis
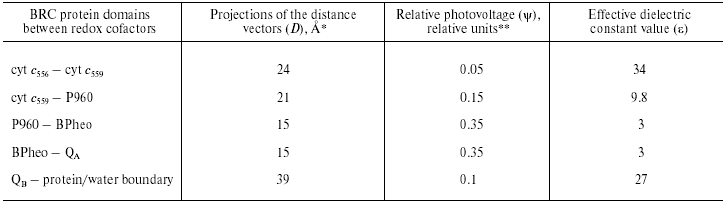
*The distances along the axis normal to the membrane plane
are given according to the X-ray data [31].
**Relative contributions of partial reactions to the overall
electrogenesis were revealed in [13] and [32].
Comparison of the distance vector projections onto the membrane normal with the relative photovoltage amplitudes in PS I and BRC demonstrated that the epsilon values corresponding to the electron transfer from the native donor proteins to P700 and P960 (or P870 in case of the other BRC species) were close to one another. Note that the electron transport reactions at the donor side of PS I and BRC share the following features of similarity: (i) Gibbs energy difference (DeltaG) between plastocyanin (Pc)/cyt c6 and P700 is close to DeltaG between cyt c2 and P870; (ii) electron transfer kinetics and proposed reaction mechanisms are very similar.
The main electrogenic step on the acceptor side of BRC is due to protonation of the doubly reduced secondary quinone QB [15]. The epsilon value in this region is ~20 [16], which is about 3 times higher than that at the acceptor region of PS I. Note also that the thermodynamic and kinetic properties of the terminal acceptors in two complexes significantly differ. The redox midpoint potential (Em) values of the QA/QA- and QB/QB- redox couples in BRC are in the range of -50 to +100 mV, whereas the Em values of FX, FA, and FB in PS I are much more negative (range of -500 to -700 mV). The lifetimes of the electron and proton transfer reactions on the acceptor side of BRC (submillisecond time range) are at least three orders of magnitude lower than those values at the domain of the iron-sulfur clusters in PS I (range of tens- to hundreds of nanoseconds). Perhaps, the three-order-of-magnitude difference between the rate constants of charge transfer on the acceptor side of BRC and PS I, and threefold increase in the estimated value of epsilon reflects the hypothetical correlation between the reaction rates and the dielectric properties of the corresponding protein domains between redox cofactors suggested in our earlier works [11, 12].
PHOTOSYSTEM II
Recently determined crystal structures of PS II core complex from the cyanobacteria Synechococcus elongatus, Thermosynechoccus vulcanus, and Thermosynechoccus elongatus [17-19] were resolved at 3.8, 3.7, and 3.5 Å resolution, respectively. It follows from these data that the arrangement of the electron transfer cofactors in PS II is rather similar to that in RCs of purple bacteria. The cofactors form two branches organized symmetrically along the pseudo-C2 axis connecting the P680 chlorophyll dimer center and nonheme iron atom. This symmetry is broken at the lumenal side of D1 protein by the redox-active Tyr161 (YZ) and the manganese cluster [Mn]4, which is located ~15 Å off the pseudo-C2 axis.
The kinetics and the quantum yield of the primary charge separation in pea chloroplasts were studied using the light-gradient technique [20, 21]. The charge separation occurred with two electrogenic phases: the faster phase with the rise-time <50 psec was ascribed to the electron transfer from the primary donor Chl (chlorophyll) dimer P680 to the intermediary acceptor pheophytin (Pheo), while the slower phase (~500 psec) was attributed to the electron transfer from Pheo to the primary quinone acceptor QA. The relative photovoltage contributions of the faster and slower phases were approximately equal to each other. Fast photovoltage measurements using PS II membranes electrically oriented in a microcoaxial cell [22] and using PS II-containing proteoliposomes attached to a planar phospholipid membrane [6, 23, 24, 26-28] showed that electron transfer from YZ to P680+ spanned a dielectrically weighted distance of 13-18% of the distance between P680 and QA [24, 25]. The dielectrically weighted distance of the electron transfer from [Mn]4 to YZox (S1-->S2 transition of the oxygen-evolving complex) was estimated to be less than 3.5% [26, 27]. Larger electrogenic components (about 7%) were attributed to proton transfer from the oxidized cofactor X to the lumen phase during the transition S2-->S3 [26].
The charge transfer reaction associated with protonation of double-reduced secondary plastoquinone QB2- at the acceptor side contributes about 5% to the overall electrogenesis in PS II [23, 24]. However, it should be noted that this estimate most probably represents the lower limit of the relative contribution of this reaction, because: (i) there were some indications that the reconstruction of the QB function in the PS II particles used in these experiments was incomplete and (ii) slow component of Deltapsi decay (characteristic of the oxygen-evolving complex activity [29]) contributed only ~50% to the overall laser flash-induced Deltapsi decay [23]. It was shown recently in our laboratory using PS II preparations, in which the contribution of the slow component of the Deltapsi decay reached ~90%, the amplitude of the electrogenic phase attributed to QB2- protonation was ~11% of total amplitude of Deltapsi [28]. The distance between the QB-binding site and protein globule boundary in PS II complexes is smaller than in BRC [19]. Therefore, it is safe to suggest that the dielectric properties in the QB-protein boundary domain of PS II are similar to that of BRC (Tables 1 and 2).
Table 2. Calculation of effective dielectric
constant values in the protein domains between redox cofactors of PS
II
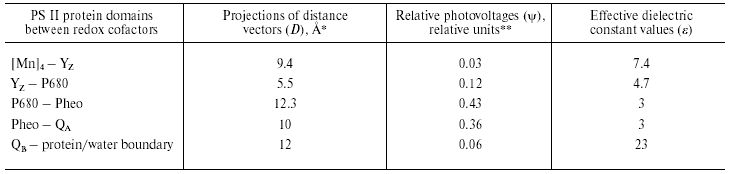
*Distances along the axis normal to the membrane plane are given
according to the atomic coordinates obtained from Protein Data Bank
(Accession codes 1FE1 [17], 1IZL [18], and 1S5L [19]).
**Relative contributions of partial reactions to the overall
electrogenesis were revealed in [22, 25-29].
The data shown in Table 2 give the results of comparison of the distance vectors between the redox cofactors in PS II with relative photovoltages accompanying corresponding charge transfer reactions. Like in the case of the BRC (see Table 1), the epsilon value in the protein domain between the Chl (bacteriochlorophyll) dimer and QA is the lowest, whereas it gradually increases at the donor side. A similar increase in the effective dielectric constant is observed at the donor side of PS I [11, 12]. Note that the epsilon value in the domain between YZ and P680 is only ~1.5 times higher than that at the P680-QA region, while the epsilon values in the Pc-P700 and cyt c559-P960 protein regions of PS I and BRC, respectively, increase about threefold relative to the hydrophobic core domains (Tables 1 and 2), which is seemingly consistent with higher electron transfer rate (30 nsec [22]) compared to that at the donor sides of PS I and BRC (microsecond-to-millisecond lifetime range). On the other hand, the values of epsilon calculated for the [Mn]4-YZ, Pc-P700, and cyt c559-P960 protein regions were larger than that for YZ-P680, and comparable with each other, which seems to be consistent with similar rate constants of corresponding electron transfer reactions.
Because similar patterns of distribution of dielectric constant are observed at least in three photosynthetic systems with different structures, it is safe to suggest that such a character of dielectric constant distribution is a general property inherent in photosynthetic charge transfer processes rather than a unique characteristic of specific pigment-protein complexes.
POSSIBLE MECHANISM OF CORRELATION BETWEEN KINETIC, THERMODYNAMIC,
AND DIELECTRIC PROPERTIES OF REACTION CENTERS
The process of charge transfer in biological systems proceeds through several stages including tunneling and further stabilization of energy levels. The efficiency of the process substantially depends on the dielectric reorganization of medium. Effective processes of relaxation (e.g., polarization) in the locus of reaction groups are required to provide stabilization of separated charges. Perhaps, the above-mentioned correlation between the rate constant of charge transfer reaction and dielectric constant in PS I complex is due to relaxation processes.
Let us consider the process of electron transfer along the chain composed of a series of several carriers A1, A2, A3, etc.:
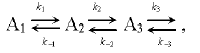 (3)
(3)where k1 and k-1 are the rate constants of direct and back transfer at the segment A1 <=> A2, respectively.
The concentration changes of the carrier A1 contains kinetic components corresponding to both direct and back reactions (k1 and k-1). However, the amplitude contribution of the kinetic component corresponding to back reaction (k-1) is determined by the ratio of the rates of direct and back transfer A2 (k2 and k-1). For example, if k2 >> k-1, an electron from A2 is rapidly transferred to A3, and the amplitude contribution of the kinetic component corresponding to the rate constant of back transfer (k-1) is small. The total rate of concentration changes of carrier A1 in this case is determined by the rate constant k1 of fast direct reaction. Otherwise, (k2 ~ k-1), the total rate of concentration changes of carrier A1 is slowed down. The negative logarithm of the rate constant of the back reaction is proportional to activation (DeltaG). At large value of DeltaG, the probability of back transfer is low, whereas this probability increases upon decreasing the DeltaG. The reaction becomes substantially reversible and the total rate of the process decreases.
The primary processes of electron transfer in photosynthesis are characterized by high rates (reaction time, from pico- to nanoseconds) and large value of DeltaG (up to 0.3 eV). As the electron goes farther from the primary pair of chlorophyll molecules, the value of DeltaG decreases and reaction time increases. Perhaps, such energy “exchange” for reaction time is of functional significance. High rate and large DeltaG of primary reactions increase the quantum yield of photosynthesis (up to ~100%) and prevent functionally useless recombination of separated charges. On the other hand, small value of DeltaG and relatively large reaction time at the peripheral segments of chain provide more effective coupling of electron transfer with metabolic and energy storage reactions (e.g., accumulation of reduced products).
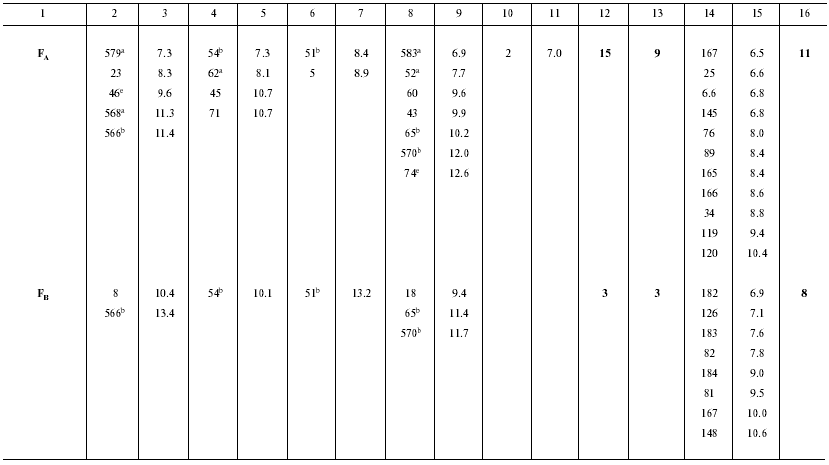
Ionizable amino acid residues and free water molecules in the vicinity of electron-transport cofactors give rise to appearance of polarization sublevels, decrease in the value of DeltaG, and increase in the dielectric constant epsilon. Let us consider the distribution of ionizable amino acid residues and free water molecules in the vicinity of electron-transport cofactors at the acceptor side of PS I by the example of the iron-sulfur centers FX, FA, and FB (Table 3).
Table 3. Distances (D) from
iron-sulfur centers to closest (D < 10 Å) polar amino
acid residues and water molecules in PS I complexes

Note: The structure file was taken from RCSB Protein Data Bank, PDB ID:
1JB0 [25]. Distance measurements were performed
using VMD 1.7.1 software (Theoretical Biophysics Group, Beckman
Institute for Advanced Science and Technology, University of Illinois).
Distance D from amino acid residue to iron-sulfur center was
measured as the shortest distance between the charged residue and the
closest iron atom of the center. Groups of residues compensating each
other are denoted as:
a ASP579, ASP568, GLU62, ARG583, ARG52; net
charge -1;
b ASP566, GLU54, LYS51, ARG570, ARG65; net charge +1;
c ASP580, ARG712; net charge 0;
d ASP593, ARG728; net charge 0;
e ASP46, ARG74; net charge 0.
In the preceding works we obtained the following estimates of epsilon distribution along the electron transfer chain in the pigment-protein complexes of PS I: epsilon ~ 5.4 (A0-->A1 and A1-->FX), epsilon ~ 8.7 (FX-->FA), and epsilon ~ 4.6 (FA-->FB) [11, 12]. These estimates of epsilon are qualitatively consistent with the pattern of distribution of water molecules and ionizable amino acid residues (Asp, Glu, Lys, Arg, and His) in the loci of the electron transfer chain. The numbers of ionizable amino acid residues in the vicinity of the iron-sulfur clusters FX, FA, and FB (at distances <11 Å from each cluster), determined from the X-ray diffraction analysis of the PS I protein crystals, are 13, 15, and 3, respectively. With regard to the effect of compensation of closely located opposite charges (distance < 3.5 Å) [30], these numbers are 5, 9, and 3, respectively. Although the general trend toward an increase in the value of epsilon along the direction from the primary pair to the periphery of the complex is valid in this case too, there is a deviation from this trend at the site FA-->FB, where distribution of water molecules and polar amino acid residues is abnormal. The cause of the anomaly is presently obscure.
Thus, BRC complexes are similar to PS I and PS II complexes not only in terms of hierarchy of electron transfer rate constants and energy gaps between neighboring carriers but also in terms of hierarchy of distribution of local effective dielectric constant. Possible mechanisms of correlation between these parameters deserve more profound experimental and theoretical research.
This study was supported by Grant from the International Science and Technology Center (ISTC) 2296 (to A. Y. S.), Grant 01-483 from the INTAS (to A. Y. S.), Grants from the Russian Foundation for Basic Research 03-04-49219 (to S. K. C.) and 03-04-48983 (to A. Y. S.), and Grant RC1-2400-MO-02 (to A. Y. S.) from the Civilian Research and Development Foundation (CRDF).
REFERENCES
1.Drachev, L. A., Jasaitis, A. A., Kaulen, A. D.,
Kondrashin, A. A., Liberman, E. A., Nemecek, I. B., Ostroumov, S. A.,
Semenov, A. Yu., and Skulachev, V. P. (1974) Nature,
249, 321-324.
2.Drachev, L. A., Kaulen, A. D., Khitrina, L. V., and
Skulachev, V. P. (1981) Eur. J. Biochem., 117,
461-470.
3.Drachev, L. A., Semenov, A. Yu., Skulachev, V. P.,
Smirnova, I. A., Chamorovsky, S. K., Kononenko, A. A., Rubin, A. B.,
and Uspenskaya, N. Ya. (1981) Eur. J. Biochem., 117,
483-489.
4.Mamedov, M. D., Gadjieva, R. M., Gourovskaya, K.
N., Drachev, L. A., and Semenov, A. Yu. (1996) J. Bioenerg.
Biomembr., 28, 517-522.
5.Vassiliev, I. R., Jung, Y.-S., Mamedov, M. D.,
Semenov, A. Yu., and Golbeck, J. H. (1997) Biophys. J.,
72, 301-315.
6.Mamedov, M. D., Lovyagina, E. R., Verkhovsky, M.
I., Semenov, A. Yu., Cherepanov, D. A., and Shinkarev, V. P. (1994)
Biochemistry (Moscow), 59, 685-690.
7.Lockhart, D. J., and Kim, P. S. (1992)
Science, 257, 947-951.
8.Steffen, M. A., Lao, K., and Boxer, S. G. (1994)
Science, 264, 810-816.
9.Finkel'shtein, A. V., and Ptitsyn, O. B. (2002)
Protein Physics [in Russian], Knizhnyi Dom Universitet,
Moscow.
10.Fersht, A. (1999) Structure and Mechanism in
Protein Science: A Guide to Enzyme Catalysis and Protein Folding,
Freeman, New York.
11.Semenov, A. Yu., Mamedov, M. D., and Chamorovsky,
S. K. (2003) FEBS Lett., 553, 223-228.
12.Semenov, A. Yu., Chamorovsky, S. K., and Mamedov,
M. D. (2004) Biofizika, 49, 227-238.
13.Dracheva, S. M., Drachev, L. A., Konstantinov, A.
A., Semenov, A. Yu., Skulachev, V. P., Arutyunian, A. M., Shuvalov, V.
A., and Zaberezhnaya, S. M. (1988) Eur. J. Biochem., 171,
253-264.
14.Semenov, A. Yu. (1991) Electrogenic Reactions
in Photosynthetic Reaction Centers of Purple Bacteria, in Soviet
Scientific Reviews/Section D (Skulachev, V. P., ed.) Vol. 10, pp.
45-75, Harwood Academic Publishers, UK.
15.Kaminskaya, O. P., Drachev, L. A., Konstantinov,
A. A., Semenov, A. Yu., and Skulachev, V. P. (1986) FEBS Lett.,
2, 224-228.
16.Shinkarev, V. P., Drachev, L. A., Mamedov, M. D.,
Mulkidjanian, A. Ya., Semenov, A. Yu., and Verkhovsky, M. I. (1993)
Biochim. Biophys. Acta, 1144, 285-294.
17.Zouni, A., Witt, H.-T., Kern, J., Fromme, P.,
Krauss, N., Saenger, W., and Orth, P. (2001) Nature, 409,
739-743.
18.Kamiya, N., and Shen, J.-R. (2003) Proc. Natl.
Acad. Sci. USA, 100, 98-103.
19.Ferreira, K. N., Iverson, T. M., Maghlaoui, K.,
Barber, J., and Iwata, S. (2004) Science, 303,
1831-1838.
20.Trissl, H.-W., and Leibl, W. (1989) FEBS
Lett., 244, 85-88.
21.Leibl, W., Breton, J., Deprez, J., and Trissl,
H.-W. (1989) Photosynth. Res., 22, 257-275.
22.Pokorny, A., Wulf, K., and Trissl, H.-W. (1994)
Biochim. Biophys. Acta, 1184, 65-70.
23.Mamedov, M. D., Beshta, O. E., Samuilov, V. D.,
and Semenov, A. Yu. (1994) FEBS Lett., 350, 96-98.
24.Hook, F., and Brzezinski, P. (1994) Biophys.
J., 66, 2066-2072.
25.Jordan, P., Fromme, P., Klukas, O., Witt, H. T.,
Saenger, W., and Krauss, N. (2001) Nature, 411,
909-917.
26.Haumann, M., Mulkidjanian, A., and Junge, W.
(1997) Biochemistry, 36, 9304-9315.
27.Mamedov, M. D., Beshta, O. E., Gourovskaya, K.
N., Mamedova, A. A., Neverov, K. D., Samuilov, V. D., and Semenov, A.
Yu. (1999) Biochemistry (Moscow), 64, 504-509.
28.Mamedov, M. D., Tyunyatkina, A. A., and Semenov,
A. Yu. (2005) Biochemistry (Moscow), in press.
29.Rutherford, W., and Boussac, A. (1992) in
Research in Photosynthesis. Proc. 9th Int. Congr. on
Photosynthesis (Murata, N., ed.), Vol. 2, pp. 21-27, Kluwer
Academic Publishers, Dordrecht, The Netherlands.
30.Antonkine, M. L., Jordan, P., Fromme, P., Krauss,
N., Golbeck, J. H., and Stehlik, D. (2003) J. Mol. Biol.,
327, 671-697.
31.Deisenhofer, J., Epp, O., Miki, K., Huber, R.,
and Michel, H. (1984) Nature, 318, 618-624.
32.Deprez, J., Trissl, H. W., and Breton, J. (1986)
Proc. Nat. Acad. Sci. USA, 83, 1699-1703.